Roof Of Implicit Function Theorem

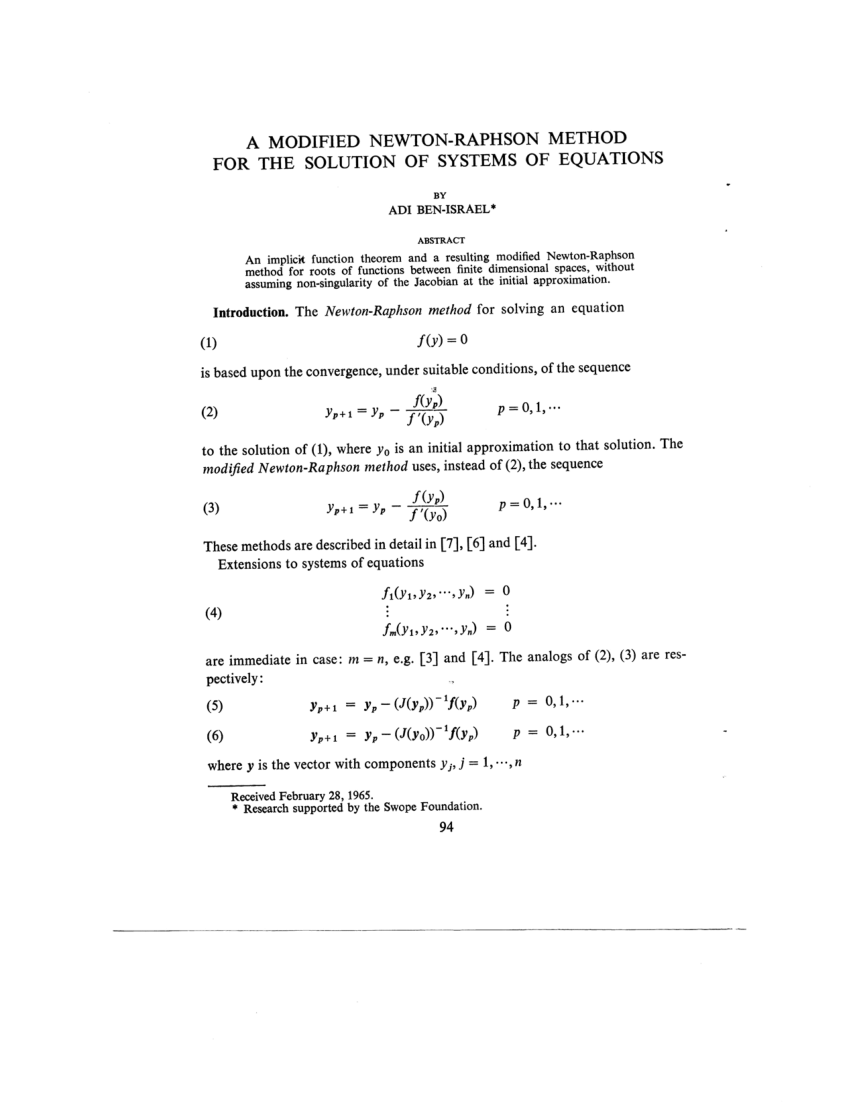
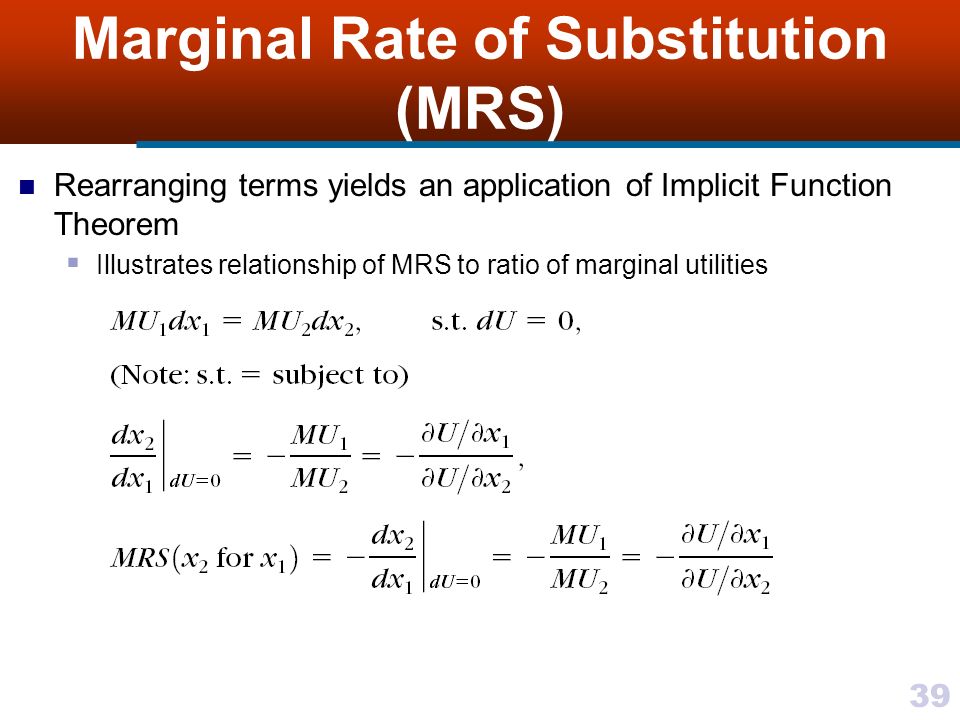
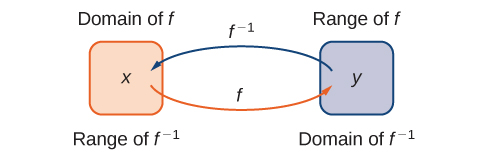
In mathematics more specifically in multivariable calculus the implicit function theorem is a tool that allows relations to be converted to functions of several real variables.

Roof of implicit function theorem.

Theorem 4 implicit function theorem. Definition 1an equation of the form. Let e rn m be open and f. Then there exists an open set u.

When profit is being maximized typically the resulting implicit functions are the labor demand function and the supply functions of various goods. This is given via inverse and implicit function theorems. Y a b 6 0. Kx ak jy bj gso that 1 for each xsuch that kx ak there is a unique ysuch that jy bj for which f x y 0.

It does so by representing the relation as the graph of a function. We also remark that we will only get a local theorem not a global theorem like in linear systems. Suppose a function with n equations is given such that f i x 1 x n y 1 y n 0 where i 1 n or we can also represent as f x i y i 0 then the implicit theorem states that under a fair condition on the partial derivatives at a point the m variables y i are differentiable functions of the x j in some section of the point. F x p y 1 implicitly definesxas a function ofpon a domainpif there is a functionξonpfor whichf ξ p p yfor allp p.

You always consider the matrix with respect to the variables you want to solve for. If you have f x y 0 and you want y to be a function of x. This document contains a proof of the implicit function theorem. R r and x 0 2r.

In our case f y 2y vanishes whenever y 0 and this happens at two points. Since we cannot express these functions in closed form therefore they are implicitly defined by the equations. The theorem says that we can make y a function of x except when f y 0. The theorem also holds in three dimensions.

So that f 2. E rm a continuously differentiable map. The implicit function theorem is a basic tool for analyzing extrema of differentiablefunctions. R3 r and a point x 0 y 0 z.

Then the implicit function theorem will give sufficient conditions for solving y 1 y m in terms of x 1 x n. The implicit function theorem for r3. Whenever the conditions of the implicit function theorem are satisfied and the theorem guarantees the existence of a function bff b r 0 bfa to b r 1 bfb subset r k such that begin equation label ift repeat bff bfx bff bfx bf0 end equation among other properties the theorem also tell us how to compute derivatives of bff. It is traditional to assume thaty 0 but not essential.

Let x 0 y 0 e such that f x 0 y 0 0 and det f j y i 6 0. The implicit function theorem says to consider the jacobian matrix with respect to u and v. The two we ve already identi ed as problems. There may not be a single function whose graph can represent the entire relation but there may be such a function on a restriction of the domain of the relation.

Then there is 0 and 0 and a box b f x y. The implicit function theorem gives a sufficient condition to ensure that there is such a. Partial directional and freche t derivatives let f. This is obvious in the one dimensional case.

Consider a continuously di erentiable function f.